Question

The function f(x) = x2 + 22x + 58 is translated 4 units to the right and 16 units up. What is the vertex form of the new function? (x – 11)2 + 58 (x + 22)2 – 121 (x + 7)2 – 47 (x – 15)2 + 94

Answer 1

C

Explanation:

Answer 2

Option C is correct.

Explanation:

The given function f(x) is:

f(x) = x^2 + 22x + 58

To find the vertex find
`((-b)/(2a) )^2` and add and subtract it from both sides of the given function

b= 22, a= 1

Putting values:

`((-b)/(2a) )^2 = ((-22)/(2(1)))^2\\=((-22)/(2))^2\\= (-11)^2\\=11^2`

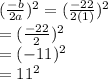
Adding (11)^2 on both sides

f(x) = x^2 + 22x + 58 +(11)^2 -(11)^2

f(x) = x^2+22x+(11)^2 +58-(11)^2

a^2 +2ab+b^2 = (a+b)^2 Using this formula:

f(x)=(x+11)^2+58-121

f(x)=(x+11)^2-63

The vertex of the given function is (-11,-63)

The function is translated 4 units to right and 16 units up

The vertex of new function will be:

(x+4,y+16) => (-11+4,-63+16)

=> (-7,-47)

So, the vertex of new function is (-7,-47)

The function will be

(x+7)^2 -47

So, Option C is correct.