Question

What is the sum of the geometric sequence 1,-6,36 if there are 6 terms

Answer 1

The sum of the six terms is 9331

Explanation:

* Lets explain what is the geometric sequence

- There is a constant ratio between each two consecutive numbers

- Ex:

# 5 , 10 , 20 , 40 , 80 , ………………………. (×2)

# 5000 , 1000 , 200 , 40 , …………………………(÷5)

* General term (nth term) of a Geometric sequence:

# U1 = a , U2 = ar , U3 = ar² , U4 = ar³ , U5 = ar^4

#
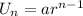`U_(n)=ar^(n-1)`, where a is the first term , r is the constant

ratio between each two consecutive terms, n is the position

of the term

- The sum of n terms of the geometric sequence is:

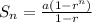`S_(n)=(a(1-r^(n)))/(1-r)` , where n is the number of the terms

a is the first term and r is the common ratio

* Lets solve the problem

∵ The geometric sequence is 1 , -6 , 36 , .........

∵ The common ratio r = U2/U1

∵ U1 = 1 and U2 = -6

∴ r = -6/1 = -6

∵ The first term is 1

∴ a = 1

∵ There are 6 terms in the sequence

∴ n = 6

∴ The sum =
`(1[1 - (-6)^(6)])/(1-6)=(1[1-46656])/(-5)=(-46655)/(-5)=9331`

* The sum of the six terms is 9331