Question

Liquid A has a vapor pressure of 264 torr at 20∘C, and liquid B has a vapor pressure of 96.5 torr at the same temperature. If 5.50 moles of liquid A and 8.50 moles of liquid B are combined to form an ideal solution, what is the total vapor pressure (in torr) above the solution at 20.0∘C?

Answer 1

Answer: 161.8 torr

Step-by-step explanation:

According to Raoult's law, the vapor pressure of a component at a given temperature is equal to the mole fraction of that component multiplied by the vapor pressure of that component in the pure state.

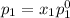`p_1=x_1p_1^0` and
`p_2=x_2P_2^0`

where, x = mole fraction

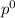`p^0` = pressure in the pure state

According to Dalton's law, the total pressure is the sum of individual pressures.

`p_(total)=p_1+p_2`
`p_(total)=x_Ap_A^0+x_BP_B^0`

`x_(A)=\frac{\text {moles of A}}{\text {moles of A+moles of B}}=(5.50)/(5.50+8.50)=0.39`,

`x_(B)=\frac{\text {moles of B}}{\text {moles of A+moles of B}}=(8.50)/(5.50+8.50)=0.61`,

`p_(A)^0=264torr`

`p_(B)^0=96.5torr`

`p_(total)=0.39* 264+0.61* 96.5=161.8torr`

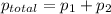
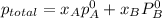
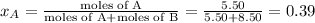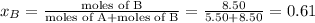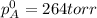
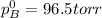
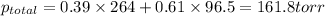
The total vapor pressure above the solution is 161.8 torr.