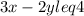Question

Which of the following inequalities matches the graph?

A.3x - 2y \geqslant 4
B.3x - 4y \leqslant 2
C.3x - 2y \leqslant 4
D.the correct inequality is not listed ​

Answer 1

C.

Explanation:

The slope-intercept form of an equation of a line:

`y=mx+b`

m - slope

b - y-intercept → (0, b)

The formula of a slope:

`m=(y_2-y_1)/(x_2-x_1)`

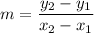
From the graph we have the points (0, -2) and (6, 7).

(0, -2) → b = -2

Calculate the slope:

`m=(7-(-2))/(6-0)=(9)/(6)=(9:3)/(6:3)=(3)/(2)`

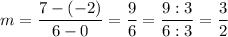
Put the value of b and m to the equation of the line in the slope-intercept form:

`y=(3)/(2)x-2`

=====================================

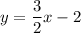
<, > - dotted line

≤, ≥ - solid line

<, ≤ - shaded region below the line

>, ≥ - shaded region above the line

======================================

We have the soli line (≤ or ≥).

Shaded region is above the line (> or ≥)

Therefore we have the answer:
`y\geq(3)/(2)x-2`

Convert to the standard form:
`Ax+By=C`

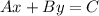
`y\geq(3)/(2)x-2` multiply both sides by 2

`2y\geq3x-4` subtract 3x from both sides

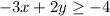`-3x+2y\geq-4` change the signs

`3x-2yleq4`