Question

The length of a rectangle is increasing at a rate of 6 cm/s and its width is increasing at a rate of 5 cm/s. When the length is 12 cm and the width is 4 cm, how fast is the area of the rectangle increasing?

Answer 1

Area of the rectangle is increasing with the rate of 84 cm/s.

Explanation:

Let l represents the length, w represents width, t represents time ( in seconds ) and A represents the area of the triangle,

Given,

`(dl)/(dt)=6\text{ cm per second}`

`(dw)/(dt)=5\text{ cm per second}`

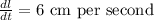
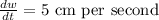
Also, l = 12 cm and w = 4 cm,

We know that,

A = l × w,

Differentiating with respect to t,

`(dA)/(dt)=(d)/(dt)(l* w)`

`=l* (dw)/(dt)+w* (dl)/(dt)`

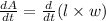
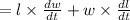
By substituting the values,

`(dA)/(dt)=12* 5+4* 6`

`=60+24`

`=84`

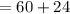
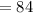
Hence, the area of the rectangle is increasing with the rate of 84 cm/s.