Answer:
domain is the set of all real numbers except 0
Explanation:
If f(x)=x-3/x and g(x)=5x-4
(f•g)(x) is f(x) times g(x)
multiply f(x) and g(x)
(f•g)(x) is f(x) times g(x)
Replace f(x) and g(x)
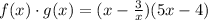
Apply FOIL method to multiply it
multiply x inside the parenthesis
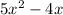
Multiply the fraction inside the parenthesis
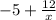
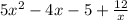
We have x in the denominator . Domain is the set of x value for which the function is defined.
When denominator x is 0 then the function is undefined
So domain is the set of all real numbers except 0