Answer: The half-life of beryllium-15 is 400 times greater than the half-life of beryllium-13.
Step-by-step explanation:
The half-life
 of a radioactive isotope refers to its decay period, which is the average lifetime of an atom before it disintegrates.
of a radioactive isotope refers to its decay period, which is the average lifetime of an atom before it disintegrates.
In this case, we are given the half life of two elements:
beryllium-13:
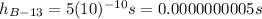
beryllium-15:
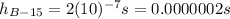
As we can see, the half-life of beryllium-15 is greater than the half-life of beryllium-13, but how great?
We can find it out by the following expression:
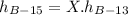
Where
 is the amount we want to find:
is the amount we want to find:
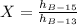

Finally:
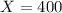
Therefore:
The half-life of beryllium-15 is 400 times greater than the half-life of beryllium-13.