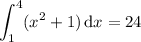Question

Estimating the area under the curve Using Wapoint sum

When rounded to the nearest hundredth, the area under the graph of f(x) = x2 + 1 from x = 1 to x = 4 with 6
subintervals using the midpoint sum is
20.38
23.94
27.88
33.47

Answer 1

Splitting up the interval [1, 4] into 6 equally-spaced subintervals gives the partition

[1, 3/2], [3/2, 2], [2, 5/2], [5/2, 3], [3, 7/2], and [7/2, 4]

each with length (4-1)/6 = 1/2.

The endpoints of each subinteval form arithmetic sequences,

`\ell_i=1+\frac{i-1}2=\frac12+\frac i2`

`r_i=1+\frac i2`

(
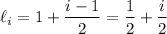
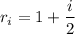
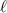`\ell` for left,
`r` for right) with
`1\le i\le6`.

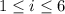The midpoints of each subinterval are, respectively,

5/4, 7/4, 9/4, 11/4, 13/4, and 15/4

which are prescribed by the sequence,

`m_i=\frac{\ell_i+r_i}2=\frac{\frac32+i}2=\frac34+\frac i2`

(
`m` for midpoint) where
`1\le i\le6`.

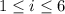The integral of
`f(x)=x^2+1` over the interval [1, 4] is approximated by the sum of the areas of rectangles with length equal to the base of each subinterval and height equal to
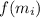`f(m_i)`, the value of
`f` at the midpoint of that subinterval:

`\displaystyle\int_1^4(x^2+1)\,\mathrm dx\approx\sum_(i=1)^6f(m_i)\frac{4-1}6=\frac12\sum_(i=1)^6\left(\left(\frac34+\frac i2\right)^2+1\right)=(383)/(16)\approx\boxed{23.94}`

Compare to the actual value of the integral,

`\displaystyle\int_1^4(x^2+1)\,\mathrm dx=24`