Answer:
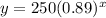
Initial value: 250
Decay
Decay rate: 11%

Initial value: 40
Growth
Growth rate: 11%
Explanation:
The function we have on the left of the table is:
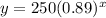
Initial value (when x = 0):
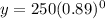
y = 250 (initial value)
Growth or Decay: 0.89 < 1 so decay
Decay rate: (1 - 0.89) * 100 = 11%
Function on right side:

Initial value (when x = 0):
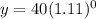
y = 40 (initial value)
Growth or decay: 1.11 > 1 so growth
Growth rate: (1.11 - 1) * 100 = 11%