Question

15 pts. Prove that the function f from R to (0, oo) is bijective if - f(x)=x2 if r- Hint: each piece of the function helps to "cover" information to break your proof(s) into cases. part of (0, oo).. you may want to use this

Answer 1

Answer with explanation:

Given the function f from R to
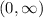
`(0,\infty)`

f:
`R\rightarrow(0,\infty)`

`-f(x)=x^2`

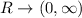
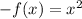
To prove that the function is objective from R to
`(0,\infty)`

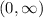
Proof:

`f:(0,\infty )\rightarrow(0,\infty)`

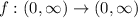
When we prove the function is bijective then we proves that function is one-one and onto.

First we prove that function is one-one

Let
`f(x_1)=f(x_2)`

`(x_1)^2=(x_2)^2`

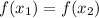
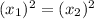
Cancel power on both side then we get

`x_1=x_2`

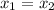
Hence, the function is one-one on domain [tex[(0,\infty)[/tex].

Now , we prove that function is onto function.

Let - f(x)=y

Then we get
`y=x^2`

`x=\sqrt y`

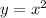
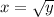
The value of y is taken from
`(0,\infty)`

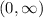
Therefore, we can find pre image for every value of y.

Hence, the function is onto function on domain
`(0,\infty)`

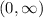
Therefore, the given
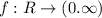`f:R\rightarrow(0.\infty)` is bijective function on
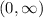`(0,\infty)` not on whole domain R .

Hence, proved.