Answer:
If there's no limits on the shape of the triangle, the length of the third side shall be between (excluding the endpoints)
- 15 kilometers, and
- 55 kilometers.
Explanation:
Let the length of the third side be
 kilometers. The length of each side shall be positive. In other words,
kilometers. The length of each side shall be positive. In other words,
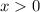 .
.
Consider the triangle inequality theorem. The sum of any two ends shall be greater than the third end. For this triangle, the lengths of the three sides are:
By the triangle inequality theorem,
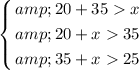 .
.
Rewrite and simplify each inequality:
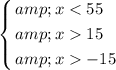
 shall satisfy all three inequalities. As a result, the range of
shall satisfy all three inequalities. As a result, the range of
 shall be the intersection of the solution sets of all three inequalities.
shall be the intersection of the solution sets of all three inequalities.
Refer to the sketch attached. On the sketch, the intersection is the region where the three colored lines are above each other. That's represents the interval
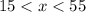 .
.
In other words, the length of the third side is supposed to be between 15 kilometers and 55 kilometers.