Question

The rate constant for the first-order decomposition of N2O5 in the reaction 2 N2O5(g)→4 NO2(g)+O2(g) is kr=3.38×10−5 s−1 at 25 °C. What is the half-life of N2O5? What will be the pressure, initially 500 Torr, after (i) 50 s, (ii) 20min after initiation of the reaction?

Answer 1

Step-by-step explanation:

`2 N_2O_5(g)\rightarrow 4 NO_2(g)+O_2(g)`

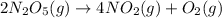
Rate of the reaction ,k=
`3.38* 10^(-5) s^(-1)`

Half life of the
`N_2O_5=t_{(1)/(2)}`

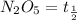
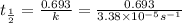`t_{(1)/(2)}=(0.693)/(k)=(0.693)/(3.38* 10^(-5) s^(-1))`(first order kinetics)

`t_{(1)/(2)}=20,502.958 seconds`

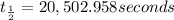
Half life of the
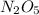`N_2O_5` is 20,502.958 seconds.

Integrated rate equation for first order kinetics in gas phase is given as:

`k=(2.303)/(t)\log(p_o)/(2p_o-p)`

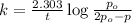
p= pressure of the gas at given time t.

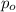`p_o` = Initial pressure of the gas

(i) When, t = 50 sec

`p_o=500 torr`

`3.38* 10^(-5) s^(-1)=(2.303)/(50 s)\log(500 Torr)/(2(500 Torr)-p)`

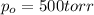
p = 500.49 Torr

(ii)When, t = 20 min = 1200 sec

`p_o=500 torr`

`3.38* 10^(-5) s^(-1)=(2.303)/(1200 s)\log(500 Torr)/(2(500 Torr)-p)`

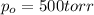
p = 519.83 Torr