Question

AB is tangent to \odot ⊙ O at A (not drawn to scale). Find the length of the radius r, to the nearest tenth.

Answer 1

r = 15.2

Explanation:

Where AB meets the circle creates a right angle. This is a right triangle problem involving missing sides. This means that we will use Pythagorean's theorem to find the length of the radius. Pythagorean's theorem applies this way:

`10^2+r^2=(r+3)^2`

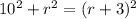
Foiling the right side gives us the equation:

`100 + r^2=r^2+6r+9`

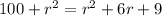
When we combine like terms, we find the squared terms cancel each other out, leaving us with

100 = 6r + 9 and

91 = 6r so

r = 15.2