Answer:
B. (5,3/4)
Explanation:
Since, when a segment having end points
 and
and
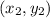 is divided by or partitioned by a point, that lies on the segment, in the ratio of m : n,
is divided by or partitioned by a point, that lies on the segment, in the ratio of m : n,
Then the coordinates of that points are,
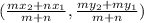
Here, point B that lies along the directed line segment from A(-5, 2) to C(11, 0) and partitions the segment in the ratio of 5:3,
Thus, the coordinates of B are,
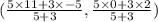

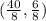
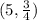
Option 'B' is correct.