Question

Choose the quadratic equation that has a leading coefficient of 1 and solutions 3 and -2.

Options:

A.) x^2+x+6=0
B.) x^2-x-5=0
C.) x^2+x+5=0
D.) x^2-x-6=0

Answer 1

Option D
`x^(2) -x-6=0`

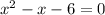
Explanation:

Verify each quadratic equation

case A) we have

`x^(2) +x+6=0`

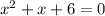
This quadratic equation has a leading coefficient of 1

Substitute the value of x=3 and x=-2 in the equation

For x=3

`(3)^(2) +(3)+6=0`

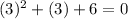
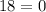`18=0` ----> is not true

therefore

x=3 is not a solution of the quadratic equation

case B) we have

`x^(2) -x-5=0`

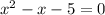
This quadratic equation has a leading coefficient of 1

Substitute the value of x=3 and x=-2 in the equation

For x=3

`(3)^(2) -(3)-5=0`

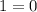`1=0` ----> is not true

therefore

x=3 is not a solution of the quadratic equation

case C) we have

`x^(2) +x+5=0`

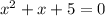
This quadratic equation has a leading coefficient of 1

Substitute the value of x=3 and x=-2 in the equation

For x=3

`(3)^(2) +(3)+5=0`

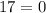`17=0` ----> is not true

therefore

x=3 is not a solution of the quadratic equation

case D) we have

`x^(2) -x-6=0`

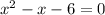
This quadratic equation has a leading coefficient of 1

Substitute the value of x=3 and x=-2 in the equation

For x=3

`(3)^(2) -(3)-6=0`

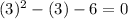
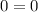`0=0` ----> is true

therefore

x=3 is a solution of the quadratic equation

For x=-2

`(-2)^(2) -(-2)-6=0`

`4+2-6=0`

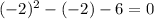
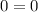`0=0` ----> is true

therefore

x=-2 is a solution of the quadratic equation