Answer:
Yes,they will intersect at (0.686,3.941) and (2.914,-2.741)
Explanation:
Given the values that represent the function g(x) you can plot them directly on a graph tool and plot the function y²+x²=16 then visualize
You can also determine the equation of the linear function as then graph both equations on the graph tool
Finding gradient m
Given (0,6) and (1,3)
m=change in value of y/change in value of x
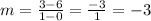
Finding the equation of the linear function
Taking points (2,0) and (x,y)

From the graph,they intersect at
(0.686,3.941) and (2.914,-2.741)