2 Answers

Alexander Doloz · Answer 1 · 2020-10-24T16:46:40+0000

Hello!

The answer is:

The correct option is:

C. 49°

To calculate the measure of the angle, we can use the following trigonometric relation:

$Cos\theta =(Adjacent)/(Hypothenuse)$

We are given the following information:

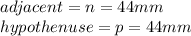
$adjacent=n=44mm\\hypothenuse=p=44mm$

So, substituting and calculating we have:

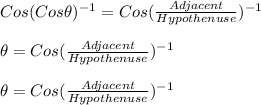
$Cos(Cos\theta)^(-1) =Cos((Adjacent)/(Hypothenuse))^(-1)\\\\\theta=Cos((Adjacent)/(Hypothenuse))^(-1)\\\\\theta=Cos((Adjacent)/(Hypothenuse))^(-1)$

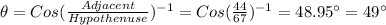
$\theta=Cos((Adjacent)/(Hypothenuse))^(-1)=Cos((44)/(67))^(-1)=48.95\°=49\°$

Hence, the correct option:

C. 49°

Have a nice day!

Teun D · Answer 2 · 2020-10-26T09:45:11+0000

Answer: Option C

49°

Explanation:

By definition, the cosine of an angle is defined as:

$cos(\theta) = (adjacent)/(hypotenuse)$ .

The hypotenuse is always the opposite side to the 90 ° angle

The adjacent side is the one that contains the angle theta and the angles of 90 °.

In this case note that:

$adjacent = n = 44\ mm\\\\hypotenuse = p = 67\ mm$

So:

$cos(\theta) = (44)/(67)$

$\theta = arcos((44)/(67))$

$\theta =49\°$