Answer: Third option
Above the dashed line
Explanation:
First we solve the inequality for the variable y.

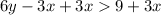


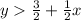
Notice that the line that limits the region is given by the equation
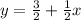
The region is formed by all the points that are greater than the points that are on the line
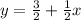 .
.
Therefore the region does not include the points that are on the line, but those that are above the line. Then the line is dashed.
The answer is the third option