Question

What are the solutions of this system of equations?

The first three steps in determining the solution set of the
system of equations algebraically are shown.
y = x2 - x-3
y=-3x + 5
(-2, -1) and (4, 17)
(-2, 11) and (4, -7)
(2, -1) and (-4, 17)
(2, 11) and (-4,-7)
Step
1
2
Equation
x– X-3 = -3x +5
0 = x² + 2x - 8
0 = (x-2)(x+4)
3

Answer 1

Answer: Third Option

(2, -1) and (-4, 17)

Explanation:

We have the following system of equations:

`y = x^2 - x-3`

`y=-3x + 5`

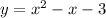
We have the first three steps to solve the system.

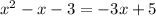`x^2- x-3 = -3x +5` equal both equations

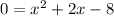`0 = x^2 + 2x - 8` Simplify and equalize to zero

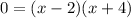`0 = (x-2)(x+4)` Factorize

Then note that the equation is equal to zero when
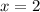`x = 2` or
`x = -4`

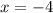
Now substitute the values of x in either of the two situations to obtain the value of the variable y.

`y=-3(2) + 5`

`y=-6 + 5`

`y=-1`

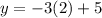
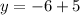
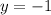
First solution: (2, -1)

`y=-3(-4) + 5`

`y=12 + 5`

`y=17`

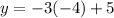
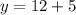
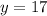
Second solution: (-4, 17)

The answer is the third option

Answer 2

Option C is correct.

Explanation:

y = x^2-x-3 eq(1)

y = -3x + 5 eq(2)

We can solve by substituting the value of y in eq(2) in the eq(1)

-3x+5 = x^2-x-3

x^2-x+3x-3-5=0

x^2+2x-8=0

Now factorizing the above equation

x^2+4x-2x-8=0

x(x+4)-2(x+4)=0

(x-2)(x+4)=0

(x-2)=0 and (x+4)=0

x=2 and x=-4

Now finding the value of y by placing value of x in the above eq(2)

put x =2

y = -3x + 5

y = -3(2) + 5

y = -6+5

y = -1

Now, put x = -4

y = -3x + 5

y = -3(-4) + 5

y = 12+5

y =17

so, when x=2, y =-1 and x=-4 y=17

(2,-1) and (-4,17) is the solution.

So, Option C is correct.