Question

Given the following equation: |4 NH3 (g) + 5 О2 (g) —> 4 NO (g) + 6 H20 () How many grams of H20 is produced if 21.1 grams of NH3 reacts with 73.9 grams of O2?

Answer 1

Answer: The mass of water that can be formed are 33.48 g.

Step-by-step explanation:

To calculate the number of moles, we use the equation:

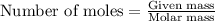`\text{Number of moles}=\frac{\text{Given mass}}{\text{Molar mass}}` ....(1)

• For ammonia gas:

Given mass of ammonia gas = 21.1 g

Molar mass of ammonia gas = 17 g/mol

Putting values in above equation, we get:

`\text{Moles of ammonia}=(21.1g)/(17g/mol)=1.24mol`

• For oxygen gas:

Given mass of oxygen gas = 73.9 g

Molar mass of oxygen gas = 32 g/mol

Putting values in above equation, we get:

`\text{Moles of oxygen gas}=(73.9g)/(32g/mol)=2.30mol`

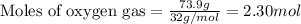
For the given chemical equation:

`4NH_3(g)+5O_2(g)\rightarrow 4NO(g)+6H_2O(l)`

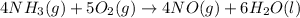
By Stoichiometry of the reaction:

4 moles of ammonia gas reacts with 5 moles of oxygen gas.

So, 1.24 moles of ammonia gas will react with =
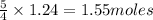`(5)/(4)* 1.24=1.55moles` of oxygen gas.

As, given amount of oxygen gas is more than the required amount. Thus, it is considered as an excess reagent.

So, ammonia gas is considered as a limiting reagent because it limits the formation of products.

By Stoichiometry of the above reaction:

4 moles of ammonia gas is producing 6 moles of water.

So, 1.24 moles of ammonia gas will produce =
`(6)/(4)* 1.24=1.86moles` of water.

Now, calculating the mass of water by using equation 1, we get:

Moles of water = 1.86 moles

Molar mass of water = 18 g/mol

Putting all the values in equation 1, we get:

`1.86mol=\frac{\text{Mass of water}}{18g/mol}\\\\\text{Mass of water}=33.48g`

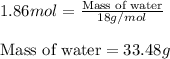
Hence, the mass of water that can be formed are 33.48 g