Answer:
Option C. 332 in²
Explanation:
In the figure attached, a regular octagon has been drawn with all equal sides and apothem OP = 10 in.
Perimeter of the given octagon is given as 66.3 in
We have to calculate the area of the octagon.
As we can see in the figure an octagon is a combination of 8 triangles.
So we will find the area of one triangle first.
Area of ΔBOC =
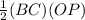
Since perimeter of octagon = 8 × one side = 8×BC
66.3 = 8× BC
BC =
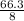
BC = 8.288 in
Therefore, area of ΔBOC =

= 5×8.288
= 41.44 in²
Now area of octagon ABCDEFGH = 8×41.44 = 331.5 ≈ 332 in²
Therefore, area of the regular octagon will be 332 in²
Option C. is the answer.