Question

If varies directly with y and x = 6 m when y = 15, find x when y = 20

Answer 1

x = 8

Explanation:

Given that x varies directly as y then the equation relating them is

x = ky ← k is the constant of variation

To find k use the condition x = 6 when y = 15

k =
`(x)/(y)` =
`(6)/(15)` = 0.4

x = 0.4y ← equation of variation

When y = 20, then

x = 0.4 × 20 = 8

Answer 2

If x varies directly with y, then :

● Increase in x results in increase of y

● Decrease in x results in decrease of y

● It is represented by : x ∝ y

`\mathsf{\bigstar\;\;If\;x\;varies\;directly\;with\;y\;then : \large\boxed{\mathsf{(x_1)/(x_2) = (y_1)/(y_2)}}}`

Here : x₁ = 6 and y₁ = 15 and x₂ = x₂ and y₂ = 20

Substituting the values we get :

`\mathsf{\implies (6)/(x_2) = (15)/(20)}`

`\mathsf{\implies x_2 = (20 * 6)/(15)}`

`\mathsf{\implies x_2 = 8}`

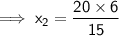
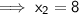
Answer : x = 8 when y = 20