Answer:
Emf through a single coil of wire is 588 V.
Step-by-step explanation:
We need to find the emf through a single coil of wire if the magnetic flux changes from -57 Wb to +43 Wb in 0.17 s
According to Faraday's law, emf of coil is directly proportional to the rate of change of magnetic flux. It can be written as :
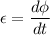
Initial flux,
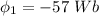
Final flux,
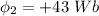
So,
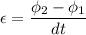

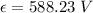
or
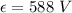
So, the EMF through a single coil of wire is 588 V. Hence, this is the required solution.