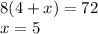Question

Leticia charges $8 per hour to babysit. She babysat Friday night for 4 hours,

and then she babysat again on Saturday. She earned a total of $72. How
many hours did Leticia babysit on Saturday?
Choose two answers: one for the equation that models this situation and one
for the correct answer.

A. Equation: 8(4 + x) = 72
B. Equation: 4(8 + x) = 72
C. Answer: 5 hours
D. Answer: 11 hours

Answer 1

Option A.

Option C.

Explanation:

Let be "x" the amount of hours Leticia babysat on Saturday.

We know that she charges $8 per hour to babysit, she babysat Friday night for 4 hours and the total amount of money she earned on those two days was $72. Knowing this, we can set up the followin equation models this situation:

`8(4+x)=72`

Finally, we must solve for "x":

`8(4+x)=72\\\\32+8x=72\\\\8x=72-32\\\\8x=40\\\\x=(40)/(8)\\\\x=5`

Answer 2

For this case we have that the variable "x" represents the number of hours that Leticia uses to take care of children on Saturday.

IF on Friday I use 4 hours ($ 8 each) and on Saturday "x" hours ($ 8 each) obtaining a profit of $ 72, we have the following equation:

`8 (4 + x) = 72`

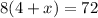
We apply distributive property:

`32 + 8x = 72\\8x = 72-32\\8x = 40\\x = \frac {40} {8}\\x = 5`

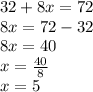
So, on Saturday she spent 5 hours.

Answer:

`8 (4 + x) = 72\\x = 5`