Question

Which of the following equations represents a line that is parallel to y=-4x-1

and passes through the point, (2, 2)?


A. y = -4x- 6
B. y = -x+2
C. y=-4x +10
D. y = -4x+4

Answer 1

For this case we have that the equation of a line of the slope-intersection form is given by:

`y = mx + b`

Where:

m: It's the slope

b: It is the cut point with the y axis.

By definition, if two straight lines are parallel then their slopes are equal.

So, if we have:

`y = -4x-1`

A parallel line has slope
`m = -4`

y = -4x + b

We substitute the point (2,2) to find "b":

`2 = -4 (2) + b\\2 = -8 + b\\b = 2 + 8\\b = 10`

Finally we have:

`y = -4x + 10`

Answer:

Option C

Answer 2

Option C : y = -4x+10

Explanation:

Given equation of line is:

`y = -4x-1`

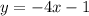
Comparing it with standard form

y=mx+b

m = -4

As the slopes of parallel lines are equal, the slop of other line will also be -4.

Now, to find the value of b

`2 = -4(2) + b\\2 = -8 + b\\2+8=b\\b=10`

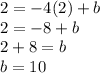
Putting the values of b and m in standard form

`y=mx+b\\y = (-4)x+10\\y= -4x+10`

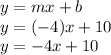
Hence,

Option C is the correct answer ..