Question

The following function describes the number of employees working at a company, in thousands, where t represents the number of years since the company revised the benefits package. f(t)=1.5(.90)^t Select the correct statement.

A. The number of employees is increasing by 50% every year.
B. The number of employees is decreasing by 10% every year.
C. The number of employees is decreasing by 90% every year.
D. The number of employees is increasing by 90% every year.

Answer 1

The answer is (B)

Explanation:

Try out each of the situations. It can't be A, since the 0.9 is less than 1, so it has to be decreasing. This leaves only B and C. Since decreasing by 90 percent is the same as multiplying by 10 percent, the only possible answer left is B.

Hope this helps!

Answer 2

the number of employees is decreasing by 10% every year

Explanation:

`f(t)=1.5(.90)^t`

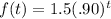
In the given function 1.5 represents the initial number of employees

Exponential function in the form of
`f(x) = a(b)^x`

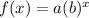
When the value of b is less than 1 then it is exponential decay

When the value of b is greater than 1 then it is exponential growth

Exponential decay factor is 0.90

`1-0.90= 0.10`

0.10 times 100 = 10%

So the number of employees is decreasing by 10% every year