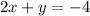Answer:
x - 2y = 4 and 2x + y = -4
Explanation:
Look at the picture.
Read the coordinates of intercepts of each line.
Put them to slope-intercept form of an equation of a line:

m - slope
b - y-intercept → (0, b)
The formula of a slope:
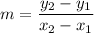
The blue line:
(4, 0), (0, -2) → b= -2

Substitute the values of a slope and an y-intercept to the equation of a line:

Convert to the standard form
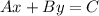 :
:
 multiply both sides by 2
multiply both sides by 2
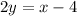 subtract x from both sides
subtract x from both sides
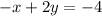 change the signs
change the signs
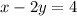
The red line:
(-2, 0), (0, -4) → b= -4
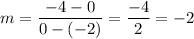
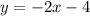 add 2x to both sides
add 2x to both sides