Question

Use the converse of the side-splitter theorem to determine if . Which statement is true?

Line segment TU is parallel to line segment RS because .
Line segment TU is not parallel to line segment RS because .
Line segment TU is parallel to line segment RS because .
Line segment TU is not parallel to line segment RS because .

Answer 1

The answer to this question is the first option:

Line segment TU is parallel to line segment RS because 32/36=40/45

Answer 2

The correct option is 1.

Explanation:

From the given figure it is clear that QT=32, TR=36, QU=40 and US=45.

The converse of side splitter theorem states that if a line divides two sides proportionally, then that line is parallel to the third side.

The ratio in which TU divides the two sides is

`(QT)/(TR)=(32)/(36)=(8)/(9)`

`(QU)/(US)=(40)/(45)=(8)/(9)`

`(QT)/(TR)=(QU)/(US)=(8)/(9)`

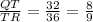
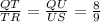
It means the line TU divides two sides proportionally.

Using converse of side splitter theorem, Line segment TU is parallel to line segment RS because

`(32)/(36)=(40)/(45)`

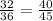
Therefore the correct option is 1.