Answer:
see explanation
Explanation:
Using the intercept form of the equation of a straight line
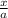 +
+
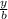 = 1
= 1
where a is the x- intercept and b the y- intercept
here a = - 2 and b = 3, hence
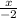 +
+
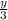 = 1
= 1
Multiply through by - 6
3x - 2y = - 6 ← equation in standard form
Substitute x = 2 and y = 6 into the left side of the equation and if equal to the right side then (2, 6) lies on the line
left side = (3 × 2) - 2(6) = 6 - 12 = - 6
Hence line passes through (2, 6)