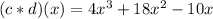Answer:
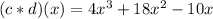
Explanation:
You know that the function
 and the function
and the function
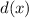 are:
are:
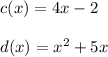
Then, in order to find
 you need to multiply the function
you need to multiply the function
 by the function
by the function
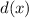 :
:
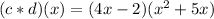
You must remember the Product of powers property, which states that:
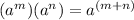
Now you can apply Distributive property:
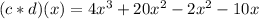
Finally, add the like terms. Then: