For this case we propose a system of equations:
x: Variable representing the plant with pink flowers
y: Variable representing the plant with purple flowers
We have as data that:
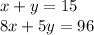
From the first equation we have to:
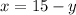
Substituting in the second equation:
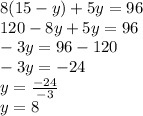
Arianna bought 8 purple flowers.
Now we have:

Arianna bought 7 pink flowers.
Answer:
Arianna bought 7 pink flowers.