Find an equation of the line perpendicular to the graph of 28x-7y=9 that passes through the point at (4,1)

Question

asked Sep 10, 2020 37.7k views

2 Answers

Grant McLean · Answer 1 · 2020-09-14T17:26:41+0000

For this case we have by definition, if two lines are perpendicular then the product of their slopes is -1.

$m_ {1} * m_ {2} = - 1$

We have the following equation:

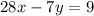
28x-7y = 9

Rewriting we have:

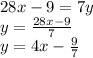
$28x-9 = 7y\\y = \frac {28x-9} {7}\\y = 4x- \frac {9} {7}$

The slope of this line is 4.

We found
$m_ {2}:$

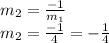
$m_ {2} = \frac {-1} {m_ {1}}\\m_ {2} = \frac {-1} {4} = - \frac {1} {4}$

The new line is of the form:

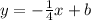
$y = - \frac {1} {4} x + b$

We substitute the given point to find the cut point "b":

$1 = - \frac {1} {4} (4) + b\\1 = -1 + b\\b = 2$

Finally, the equation is:

$y = - \frac {1} {4} x + 2$

Answer:

$y = - \frac {1} {4} x + 2$