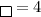ANSWER
EXPLANATION.
The given equation is
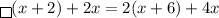
Let us expand the right hand side to obtain:

We group similar terms and keep the expression with the box on the left.
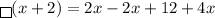
Simplify
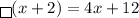
We can see that the value of box the will be the equation to have no solution is 4.
Let us substitute 4 for box.

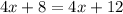
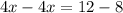
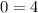
This is not true. Hence the equation has no solution when