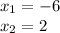Question

What are the solutions to x^2 + 4x - 12 = 0?

Answer 1

That can be factored:

(x +6) * (x -2)

Therefore, x = -6 and x =2

Explanation:

Answer 2

For this case we have the following quadratic equation:

`x ^ 2 + 4x-12 = 0`

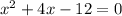
We can factor the equation, for it, we must find two numbers that when multiplied give as result -12 and when summed give as result +4. These numbers are: +6 and -2.

Then, the factorization is given by:

`(x + 6) (x-2) = 0`

Thus, the solutions are:

`x_ {1} = - 6\\x_ {2} = 2`

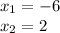
Answer:

`x_ {1} = - 6\\x_ {2} = 2`