Question

Consider a standard deck of 52 playing cards, a randomly selected card from the deck, and the following events: R = red, B = black, A = ace, N = nine, D = diamond, and C = club.

Are D and C mutually exclusive?

Answer 1

Events D and C are mutually exclusive.

Explanation:

Two events D and C are mutually exclusive if

`Pr(D\cup C)=Pr(D)+Pr(C)`

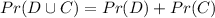
Find these probabilities. In a standard deck of 52 playing cards there are 13 diamond cards and 13 club cards.

The probabilty of chosing diamond is

`Pr(D)=(13)/(52)=(1)/(4)`

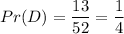
The probabilty of chosing club is

`Pr(C)=(13)/(52)=(1)/(4)`

The probabilty of chosing diamond or club is

`Pr(D\cup C)=(13+13)/(52)=(1)/(2)`

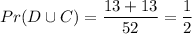
Since

`(1)/(2)=(1)/(4)+(1)/(4),`

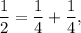
events D and C are mutually exclusive.