Question

Which values are within the range of the piecewise-defined function?

F(x)={2x+2,x<-3{x,x=-3{-x-2,x>-3

Answer 1

y=-6

y=-4

y=-3

y=0

Explanation:

Answer 2

Answer: y < 1

Explanation:

`\begin{array}c\underline{x; x<-3}&\underline{y=2x+2}&\\-5&2(-5)+2=-8&\\-4&2(-4)+2=-6&\\-3&2(-3)+2=-4&\text{approaching but not including -4}\\&&&\underline{x; x=-3}&\underline{\qquad y=x\qquad}&\\-3&-3&\\&&&\\\underline{x; x>-3}&\underline{y=-x-2}&\\-3&-(-3)-2=1&\text{approaching but not including 1}\\-2&-(-2)-2=0&\\-1&-(-1)-2=-1&\end{array}`

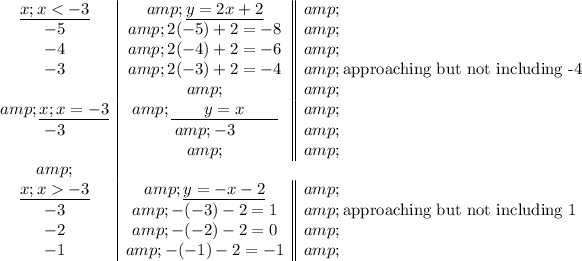
The first function has the range of y < -4

The second function has the range of y = -3

The third function has the range of y < 1

The largest y-value is 1 and the smallest y-value is -∞, therefore the range (y-values) are from -∞ to 1 → y < 1