Question

Factor completely.

-2k - k 3 - 3k 2

a.) k(-k + 1)(k - 2)
b.) -k(k - 1)(k - 2)
c.) -k(k + 1)(k + 2)

Answer 1

Option c.

Explanation:

The given expression is

`-2k-k^3-3k^2`

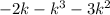
We need to find the factor form of the given expression.

Taking out HCF.

`-k(2+k^2+3k)`

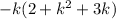
Arrange the terms according to there degree.

`-k(k^2+3k+2)`

Splitting the middle terms we get

`-k(k^2+2k+k+2)`

`-k((k^2+2k)+(k+2))`

`-k(k(k+2)+(k+2))`

`-k(k+1)(k+2)`

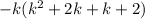
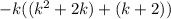
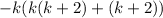
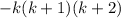
The factor form of given expression is -k(k+1)(k+2). Therefore, the correct option is c.

Answer 2

-k (k+1) (k+2)

Explanation:

-2k - k³ - 3k² (factor-k out)

-k (2 + k² + 3k) (rearrange to standard quadratic form)

-k (k² + 3k + 2) (factor expression inside parentheses using your favorite method)

-k (k+1) (k+2)