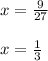Question

What is the value of x if 9x^-1 - 2 = 25

Answer 1

C on edg

Explanation:

Answer 2

Answer:
`x=(1)/(3)`

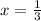
Explanation:

You need to remember the Negative exponent rule:

`a^(-n)=(1)/(a^n)`

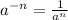
Then, having the equation
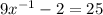`9x^(-1) - 2 = 25`, you can rewrite it in this form:

`(9)/(x) - 2 = 25`

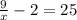
Now add 2 to both sides of the equation:

`(9)/(x) - 2+2 = 25+2\\\\(9)/(x)=27`

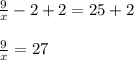
Multiply both sides of the equation by "x":

`(x)((9)/(x))=27(x)\\\\9=27x`

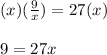
And finally divide both sides of the equation by 27.

The value of "x" is:

`x=(9)/(27)\\\\x=(1)/(3)`