Answer:
To maximize the income should be 28 buses and 160 cars
Explanation:
Let
x-----> the number of cars
y ----> the number of bus
we know that
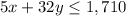 ------> inequality A
------> inequality A
 ----> inequality B
----> inequality B
The function of the cost to maximize is equal to
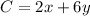
Solve the system of inequalities by graphing
The solution is the shaded area
see the attached figure
The vertices of the solution are
(0,0),(0,53),(160,28),(189,0)
Verify
(0,53)

(160,28)
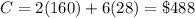
therefore
To maximize the income should be 28 buses and 160 cars