Answer: Option A

Explanation:
The javelin will have reached its maximum horizontal distance when it touches the ground.
Then the maximum horizontal distance occurs when the height h (x) is equal to zero.
So we must equal h(x) to zero and solve the equation for x.
Therefore the form that is most useful to determine the horizontal distance that the javelin covers is the one that is factored. Because it allows us to find the zeros of the quadratic function more easily

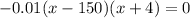
The equation is equal to zero when
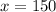 or when
or when
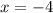
Therefore the solution is
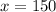 .
.
The horizontal distance that covers the javelin is 150 feet