Question

The base of a 11 foot ladder is 2 feet from a building . If the ladder reaches the flat root , how tall is the building ?

Answer 1

We can utilize the Pythagorean theorem to answer this.

11 is the hypotenuse in this case, and 2 is one of the legs.

11² = 2² + x² =

121 = 4 + x²

117 = x²

√117 = x

Answer = √117

Answer 2

Answer:
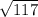`√(117)` feet or about 10.82 feet.

Explanation:

For the given situation , the ladder meeting the ground and the building is making right triangle with them [∵ building is standing vertical to the ground.]

Then, by Pythagoras theorem of right triangles , we have

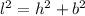`l^2=h^2+b^2` (1), where l is ladder's height ,l h is building's height and b is the base .

Given : The base of a 11 foot ladder is 2 feet from a building .

i.e. l = 11 foot and b = 2 foot

Substitute the values in the above formula (1) we get

`(11)^2=h^2+(2)^2\\\\\Rightarrow\ 121=h^2+4\\\\\Rightarrow\ h^2=121-4\\\\\Rightarrow\ h^2= 117\\\\\Rightarrow\ h=√(117)=10.8166538264\approx10.82 \text{ feet}`

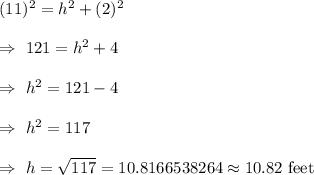
Hence, the height of building is
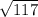`√(117)` feet or about 10.82 feet.