Question

At which values of X does the function F(x) have a vertical asymptote? Check all that apply.

Answer 1

A. 1

D. 8

Step-by-step explanation

The vertical asymptote occurs at where the denominator of a rational function in its simplest form is equal to zero.

The rational function given is

`p(x) = (9)/((x - 1)(x - 8))`

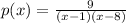
This rational function is in it's simplest form.

The vertical asymptotes occurs at

`(x - 1)(x - 8) = 0`

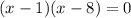
By the zero product principle, we must have either

`(x - 1) = 0 \: \: or \: \: (x - 8) = 0`

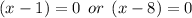
This implies that

`x =1\: \: or \: \: x = 8`

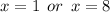
Options A and D are correct.