Answer: The width is 12 feet shorter than the length.
Explanation:
The area of a rectangle can be calculated with this formula:
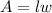
Where "l" is the lenght and "w" is the width of the rectangle.
We know that lenght of Jennie's yard is 30 feet, the width is
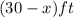 and the area is 540 square feet. Then:
and the area is 540 square feet. Then:

Substitute these values into the formula and then solve for "x":
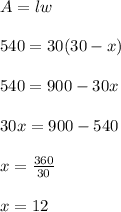
Therefore, the width is 12 feet shorter than the length.