Question

F(x)=x^2-14x+33 enter the quadratic function in factored form

Answer 1

`F(x)=(x-11)(x-3)`

Explanation:

we have

`F(x)=x^(2) -14x+33`

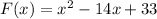
Find the zeros of the function

F(x)=0

`0=x^(2) -14x+33`

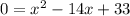
Group terms that contain the same variable, and move the constant to the opposite side of the equation

`-33=x^(2) -14x`

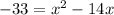
Complete the square. Remember to balance the equation by adding the same constants to each side

`-33+49=x^(2) -14x+49`

`16=x^(2) -14x+49`

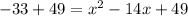
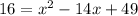
Rewrite as perfect squares

`16=(x-7)^(2)`

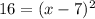
square root both sides

`(x-7)=(+/-)4`

`x=(+/-)4+7`

`x=(+)4+7=11`

`x=(-)4+7=3`

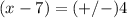
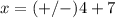
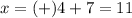
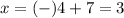
so

The factors are

(x-11) and (x-3)

therefore

`F(x)=(x-11)(x-3)`