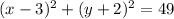Answer:
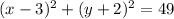
Explanation:
The center-radius form of the equation of a circle is in the format;
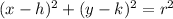
with the center being at the point (h, k) and the radius being r units.
We simply plugin the values of the center and radius given in order to determine the equation of the circle;
The equation of the circle with center (3, -2) and radius 7 is;