Question

Solve the equation for all real solutions 20p^2+33p+16=6​

Answer 1

First you must bring 6 to the other side of the equation so it equals zero. To do this subtract 6 to both sides

20p^2+33p+16 - 6=6​ - 6

20p^2+33p+10=0

Use the quadratic equation to solve this (image of the quadratic equation is below)

Remember that quadratic functions are set up like so:

`ax^(2) +bx +c = 0`

That means that in this equation...

a = 20

b = 33

c = 10

^^^Plug these numbers into the quadratic equation and solve...

`\frac{-33+/-\sqrt{(33)^(2)-4(20)(10)}}{2(20)}`

`(-33 +/-√(1089-800))/(40)`

`(-33+/-√(289) )/(40)`

`(-33+/-17)/(40)`

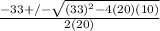
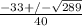
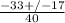
Keep in mind that +/- is ±

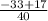`(-33+17)/(40)` ---> -2/5 ---> -0.4

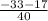`(-33-17)/(40)` ---> -5/4 ---> - 1.25

Hope this helped!

~Just a girl in love with Shawn Mendes