Question

Which quadratic function has Vertex (-1,4) and passes through (4,19)
HELP

Answer 1

`f(x)=(3)/(5)(x+1)^2+4`

Explanation:

The equation of a quadratic function in vertex form is given by:

`f(x)=a(x-h)^2+k`

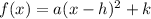
Where (h,k) is the vertex.

It was given in the question that the vertex of the parabola is (-1,4).

When we substitute the vertex into the formula we get:

`f(x)=a(x+1)^2+4`

The parabola also passes through (4,19) hence it must satisfy its equation.

`19=a(4+1)^2+4`

`19-4=a(5)^2`

`15=25a`

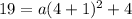
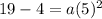
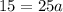
We divide both sides by 25 to get:

`a=(15)/(25)= (3)/(5)`

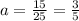
Hence the quadratic function is:

`f(x)=(3)/(5)(x+1)^2+4`