2 Answers

Assafs · Answer 1 · 2020-07-19T04:23:17+0000

Answer: The radius of the given circle is 4 units.

Step-by-step explanation: We are given to find the radius of the circle with the following equation :

x^2+y^2-10x+6y+18=0~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(i)

We know that

the standard equation of a circle with center at the point (h, k) and radius r units is given by

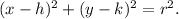
(x-h)^2+(y-k)^2=r^2.

From equation (i), we have

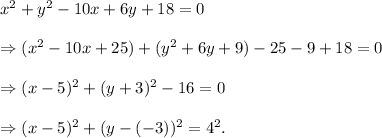
$x^2+y^2-10x+6y+18=0\\\\\Rightarrow (x^2-10x+25)+(y^2+6y+9)-25-9+18=0\\\\\Rightarrow (x-5)^2+(y+3)^2-16=0\\\\\Rightarrow (x-5)^2+(y-(-3))^2=4^2.$

Comparing it with the standard equation, the radius of the circle is given by

r=4.

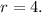
Thus, the radius of the given circle is 4 units.

Please log in or register to add a comment.