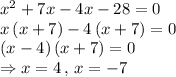Question

Which is a solution to the equation?
(x-2)(x + 5) = 18

Answer 1

Solution of the given equation:
`x=4 , -7`

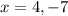
Explanation:

An equation is a mathematical statement that states that two things are equal to each other.

A linear equation in one variable is of the form
`ax^2+bx+c=0` where a,b,c are coefficients and x is variable .

Here. given:
`(x-2)(x+5)=18`

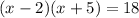
Using rule (Multiplication is distributive over addition) :
`a(b+c)=ab+ac` ,

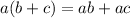we can write this equation as,

`(x-2)(x+5) =18\\ x(x+5)-2(x+5) =18\\ x^2+5x-2x-10 =18\\ x^2+3x-10-18 =0\\ x^2+3x-28 =0\\`

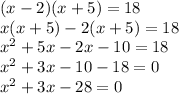
On comparing this equation with
`x^2` - (sum of roots)
`x`+(product of roots) = 0, we get

sum of roots=3 (7-4)

product of roots = -28
`\left ( 7* -4 \right )`

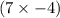
Using this fact , we can write this equation as ,

`x^2+7x-4x-28=0\\x\left ( x+7 \right )-4\left ( x+7 \right )=0\\\left ( x-4 \right )\left ( x+7 \right )=0\\\Rightarrow x=4\,,\,x=-7`

Answer 2

Hello!

Answer:

`\boxed{x=4, x=-7}\checkmark`

Explanation:

First, expand.

`x^2+3x-10=18`

Then, subtract by 18 both sides.

`x^2+3x-10-18=18-18`

Simplify and solve.

`x^2+3x-28=0`

Therefore, the solution to this equation is x=4, and x=-7.

x=4 and x=-7 is the correct answer.

Hope this helps you!

Have a nice day! :)

Thank you!