Answer: Option B
A reflection about the x-axis, a vertical stretch by a factor of 2, a horizontal shift of 1 unit to the right, and a vertical shift downward of 6 units.
Explanation:
If the graph of the function
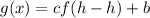 represents the transformations made to the graph of
represents the transformations made to the graph of
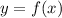 then, by definition:
then, by definition:
If
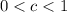 then the graph is compressed vertically by a factor c.
then the graph is compressed vertically by a factor c.
If
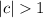 then the graph is stretched vertically by a factor c
then the graph is stretched vertically by a factor c
If
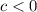 then the graph is reflected on the x axis.
then the graph is reflected on the x axis.
If
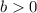 the graph moves vertically upwards b units.
the graph moves vertically upwards b units.
If
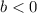 the graph moves vertically down b units
the graph moves vertically down b units
If
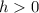 then the graph of f(x) moves horizontally h units to the left
then the graph of f(x) moves horizontally h units to the left
If
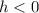 then the graph of f(x) moves horizontally h units to the right
then the graph of f(x) moves horizontally h units to the right
In this problem we have the function
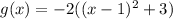 and our parent function is
and our parent function is
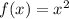
therefore it is true that
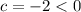 and
and
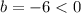 and
and
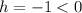
Therefore the graph is reflected on the x axis, stretched vertically by a factor 2. The graph of f(x) moves horizontally 1 units to the right and shift downward of 6 units.
The answer is (B) A reflection about the x-axis, a vertical stretch by a factor of 2, a horizontal shift of 1 unit to the right, and a vertical shift downward of 6 units.